Continuous Waveforms and Damping
A sine wave is a waveform generated by a system that is characterised by simple harmonic motion. An ideal system which exhibits simple harmonic motion is a system that loses no energy (or has its energy replenished from outside the system). Such a waveform can also be called a continuous waveform as it continues forever without eventually reducing to zero intensity.
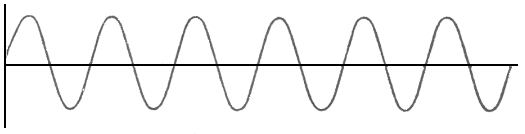
Figure 1: A continuous waveform. A sound wave exhibiting these characteristics would be a pure tone. Such a waveform would be generated by a system exhibiting ideal simple harmonic motion.
Real systems are never ideal. All naturally-occuring systems loose energy (eg. as heat due to friction). A tuning fork doesn't ring forever. Its intensity decreases over a relatively long period (say about half a minute). A tuning fork loses energy as heat (both internally as a consequence of heat loss during physical deformation and externally as a consequence of friction with air). This loss of energy in an oscillating system is know as damping. A damped waveform is also know as a non-continuous waveform.
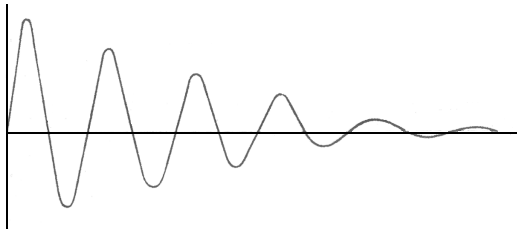
Figure 2: A non-continuous or damped waveform. A damped waveform's intensity dies out either gradually or quickly.
A damped waveform can die out quickly or slowly. A waveform that dies out quickly is said to be strongly damped as it loses energy quickly. A waveform that dies out slowly is said to be weakly damped as it loses energy slowly. The terms weakly damped and strongly damped are clearly relative terms. When compared to speech sounds, a tuning fork is said to be weakly damped as it dies down very slowly relative to each of the glottal pulses generated by the vocal folds during phonation (glottal pulses die out in a fraction of a second).
Damping is not just a characteristic of systems that generate non-continuous sine wave like patterns (as in figure 2). Damping is a characteristic of systems that produce sounds with very complex spectral patterns. If you hit your fist on a wooden table it will produce a "bang" that dies out very rapidly. The "bang" possesses a very complex aperiodic or random spectrum that dies out in less than a second and so the sound and the system generating it are much more highly damped than a tuning fork and the sound it produces. Note that it is common to refer to both a sound and the system that generates it as possessing some relative degree of damping.
Content owner: Department of Linguistics Last updated: 12 Mar 2024 9:49am