Basic acoustics
Basic Acoustics
These notes are divided into two parts. The first part, or main body of the notes, provides some basic information on acoustics. This is supplemented by explanatory footnotes which contain extra information which might aid in your understanding of the main text. The second part of these notes are the appendices which contain detailed information that you might wish to examine if you are interested.
These notes do not attempt to cover basic issues such as the nature of sound itself. During the lectures you will be referred to various references that should adequately cover such topics. These notes particularly focus on sound "amplitude", the velocity of sound, basic units and measurements of sound and the calculation of resonant frequencies.
Some Notes on Terminology
If you choose to read about these topics further in other publications, be especially careful about terminology as it tends to vary somewhat from one text to another. In this course I have chosen to use the word "AMPLITUDE" when referring generically to the concept that includes Power, Intensity and Pressure (1).
It is common for engineers to talk about "POWER" when discussing sound amplitude. Physicists will tell you that Power is the total work done per second by a sound source. What the engineers are often referring to when they use the word "power", however, is acoustic "INTENSITY" or power per unit area (ie. per square metre). The terms "power" and "intensity" are used interchangeably by many authors. For this course we will use the term "intensity" in preference to the term "power" unless we are explicitly discussing the total acoustic power output of a sound source.
What is measured directly at a microphone diaphragm is "PRESSURE" or "SOUND PRESSURE LEVEL" (SPL) which is a measure of the slight fluctuations in the ambient pressure of the medium (eg. air) through which the sound is being conducted (2). Once you have derived a pressure value you can then mathematically convert that into an intensity value.
In these notes the following symbols have been used:-
| Power | Pwr |
| Intensity | I |
| Pressure | P |
| Sound Pressure Level | SPL |
BEWARE: It is very common to find the symbol "P" referring to "Power" in many publications. Be 100% sure as to whether "P" refers to Power or Pressure in any publication you are reading. This is particularly important when engineers use "Power" to refer to "Intensity" and so use the symbol "P" instead of "I" in their formulae. This usage has the potential to greatly confuse you, especially in dB formulae (ie. what is really an Intensity-to-dB formula may seem to you to be a Pressure-to-dB formula).
Acoustic Units of Measurement (3)
The main units of measurement of relevance to acoustics are as follows (described in detail elsewhere):-
| Name | Abbreviation | Basic Units (4) | (non-Basic Units) | |
1) Wavelength (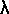 ): ):
| metre | m | m | |
| 2) Frequency (f): |
Hertz kiloHertz |
Hz kHz | s-1 |
(cycles.s-1) (~) (1000.s-1) |
| 3) Period (T): |
second millisecond |
s ms | s | (10-3.s) |
| 4) Speed of sound (c): | metre/sec | m/s | m.s-1 | |
| 5) Acoustic Power (Pwr): | Watt | W | kg.m2.s-3 | (Joule.s-1) |
| 6) Acoustic Intensity (I): | Watt/sq.m. | W/m2 | kg.s-3 | (Joule.s-1.m-2) |
| 7) Sound Pressure (P, SPL): |
Pascal microPascal |
Pa µPa (uPa) | kg.m-1.s-2 |
(Newton.m-2) (10-6.Pa) |
Wavelength, Period, Frequency and the Speed of Sound (5): Definitions and Conversions
The wavelength (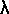 ) of a wave is the distance between successive wave fronts (ie. peak-to-peak distance). Wavelength is measured in metres (m).
) of a wave is the distance between successive wave fronts (ie. peak-to-peak distance). Wavelength is measured in metres (m).
The frequency (f) of a wave is the number of times per second that a complete wave cycle passes an observer. Frequency is measured in Hertz (Hz) {or /second (s-1) in basic units}.
The period (T) of a wave is the time it takes for one wave cycle to pass an observer. The period is measured in seconds (s) (in speech milliseconds (ms) are commonly used)
The speed or velocity of sound (c) is the number of metres that a wave front can travel in a second. The speed of sound is measured in metres/second (m.s-1)
From the above it can be seen that the period and frequency of a wave are the inverse of each other:-
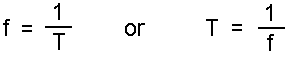
Before making such calculations be sure that the values are in Hz and seconds. Milliseconds (ms) can be converted to seconds by dividing by 1000. KiloHertz (kHz) can be converted to Hz by multiplying by 1000.
Examples:
i) Calculate the period of a wave that has a frequency of 1 kHz. (convert to 1000 Hz)
T = 1 / 1000 = 10-3 s ( = 1 ms )
ii) Calculate the frequency of a wave with a period of 10 ms. (convert: 10/1000 = 0.01 s)
f = 1 / 0.01 = 100 Hz
It is possible to calculate the frequency of a wave if you know its wavelength and the speed of sound. Conversely, you can calculate the wavelength of a wave from its frequency and the speed of sound. (Similarly, for period (T)).
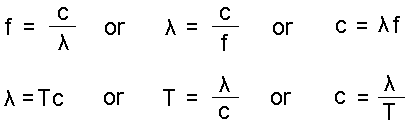
It should be clear from this that if the speed of sound changes then there will be a change in the apparent resonant characteristics of a resonator. For example, if the speed of sound increases the frequency of a sound emanating from a resonator will increase.
The speed of sound varies greatly from one medium to another. For the purposes of this course, the speed of sound in liquids and solids will be ignored. Speech sounds are normally transmitted through gases and this is almost always normal air.
At 1 atm. pressure and 0°C the speed of sound in air is 331 m.s-1. This value is sometimes rounded down to 330 m.s-1 (to simplify calculations) or rounded up to 333. (so that the speed of sound can be quoted as travelling 1 Km in 3 seconds).
The speed of sound is proportional to the temperature and inversely proportional to the density of the gas through which it travels:-
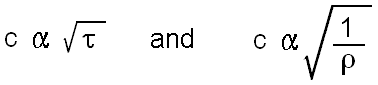
| where: | 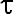 is the Absolute temperature in °K ( 0°C = 273°K) is the Absolute temperature in °K ( 0°C = 273°K)
|
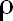 is the density of the gas is the density of the gas
|
The only other gases in which a speech researcher might encounter speech is in pure oxygen (usually in medical applications) and helium/oxygen (Heliox) mixtures used in deep sea diving. Pure oxygen (slightly denser than air) has a speed of sound which is slightly lower than for normal air, whilst the speed of sound in Heliox mixtures is considerably higher than for normal air, owing to the very much lower density of helium when compared to nitrogen (the gas normally found with oxygen in air). The exact speed of sound in Heliox mixtures depends upon the ratio of helium to oxygen, but is around twice the speed of sound in normal air. As we saw above, when the speed of sound is changed the apparent resonant characteristics of sounds emitted by an acoustic resonator (such as the vocal tract) are changed. In the case of speech in Heliox, the fundamental frequency (pitch) and resonant peak (formant) frequencies are shifted to much higher values (approximately doubled) to give the speech of deep sea divers a "chipmunk" sound.
It should also be clear that as temperature increases then so will the speed of sound. It is most important to note that temperature is Absolute Temperature in K and so the ratio of:-
2°C / 1°C 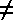 2 2
| |
| Rather | 275K / 274K = 1.00365 |
What this means is that the effect of temperature change on the speed of sound within temperature ranges at which speech is normally uttered (say: -50°C to +45°C) only results in moderate changes in the speed of sound.
Change in the speed of sound resulting from changes in temperature can be derived from the following formula:-
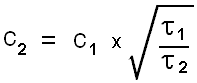
| where | c1 and 1 are the speed of sound in and temperature (°K) of condition 1 |
| and c2 and 2 are the speed of sound in and temperature (°K) of condition 2 |
For example, c at -50°C is 84% of c at +45°C, which would result in perceivable differences in the frequency of the same sound. (See appendix 6 for a further example)
Sound "Amplitude"
The human ear and the microphone (the main artificial transducer of sound) both measure the tiny changes in pressure that result from the passage of a longitudinal wave through a medium.
The average air pressure at sea level is approximately equivalent to the pressure exerted by a column of mercury 76 cm high (in a barometer) at 0°C under standard gravity. This approximation has been adopted as the definition of the standard measure of air pressure, 1 atmosphere (atm). This unit of measurement is is equivalent to (6):-
1 atm  1.013 x 105 Pa
1.013 x 105 Pa
The sound pressure that is only just perceivable (ie. the threshold of hearing for a 1000 Hz tone) is taken to be (7):-
| 2 x 10-5 Pa (ie. 20 µPa) | Standard Reference Sound Pressure Level |
nb. the actual threshold of hearing varies greatly from frequency to frequency as well as from person to person. (This value is actually a better approximation of threshold at 3200 Hz.)
Note that the actual sound pressure involved is about 2 x 10-10 times (ie. 1/5,000,000,000) atmospheric pressure. It is common to quote sound pressure in µPa as this measure is almost of the same order of magnitude as the minimum perceivable sound pressures.
The threshold of pain (ie. the maximum sound pressure that can be perceived without pain) is about 100 Pa or about 1/1000 atm, which is 5,000,000 times the threshold sound pressure.
Thresholds have also been defined in terms of intensity, with the standard intensity threshold of hearing being (8):-
| 10-12 Watts.m-2 | Standard Reference Sound Intensity |
The intensity of a sound, with a sound pressure level of 20 µPa, is very close to 10-12 Watts.m-2. These two reference values have been rounded off and so do not describe precisely the same sound, but they are very close.
The intensity of a sound is proportional to the square of the sound pressure.
I  P2
P2
The following is approximately true for sound travelling through air at 1 atm and 20°C:-
I  (P/20)2
(P/20)2
The ratio of the intensity of sound #1 (I1) over the intensity of sound #2 (I 2) equals the square of the ratio of the sound pressure of sound #1 (P1) over the sound pressure of sound #2 (P 2). (nb. P1 is the sound pressure of a sound when that sound's intensity is I 1, and P 2 is the sound pressure of a sound when that sound's intensity is I 2).
I1/I2 = (P1/P2)2
deciBels (dB)
It was noted many years ago (Fechner, 1860) that the sensitivity of the ear to changes in intensity was not related linearly to either intensity or pressure. It was believed then that the ear's sensitivity to sound intensity or sound pressure was an approximately logarithmic relationship. Initially, it was proposed that a new measure of intensity be utilised which was derived from the log (base 10) of the ratio of two intensities.
Bel (IL) = log10 (I1/I2)
The Bel scale (named after Alexander Graham Bell) was approximately linearly related to the ear's sensitivity to sound intensity (at intensities louder than a whisper) so that equal steps in Bels were close to equal perceptual steps. A step of 1 Bel was however about 10 times greater than the minimally perceivable step and so a new scale was devised, the deciBel (dB).
1 dB = 0.1 Bel or 1 Bel = 10 dB
So that:-
dB = 10 x log10 (I1/I2)
Note that it is common to refer to dB values as "Intensity in dB" (whether derived from intensity or sound pressure) (9).
If I2 is set to a standard reference intensity then dB measurements become readily comparable from one publication to another. The usual reference level chosen is the Standard Reference Sound Intensity of 10-12 Watts.m-2 (see previous page) and is indicated by the symbol "I0". Such dB values are referred to as dB (IL) to indicate that the dB value has been determined from the ratio of the sound's intensity to the standard reference intensity.
dB (IL: ref=10-12 W.m-2) = 10 x log10 (I/I0)
DeciBel values can also be derived directly from sound pressure (conveniently, as this is the way sound amplitude is measured by a microphone) (10). As with intensity, dB can be calculated from the ratio of any two sound pressures (P1 and P2):-
dB = 20 x log10(P1/P2)
If P2 is set to a standard reference sound pressure then dB measurements become readily comparable from one publication to another. The usual reference level chosen is the Standard Reference Sound Pressure of 20µPa (see previous page) and is indicated by the symbol "P0". Such dB values are referred to as dB (SPL) to indicate that the dB value has been determined from the ratio of the sound's sound pressure level to the standard reference sound pressure.
dB (SPL: ref=20µPa) = 20 x log10(P/P0)
It is conventional to indicate whether dB was calculated from intensity or pressure mainly because of the slight difference in the reference levels (which could result in significant differences in the resultant dB value for very low test intensities and pressures). This is usually indicated in one of the following ways:-
| dB (IL) | = | 10 x log10(I/I0) |
| dB (IL: ref=10-12W.m-2) | = | 10 x log10(I/I0) |
| dB (ref=10-12W.m-2) | = | 10 x log10(I/I0) |
| dB (SPL) | = | 20 x log10(P/P0) |
| dB (SPL: ref=20µPa) | = | 20 x log10(P/P0) |
| dB (ref=20µPa) | = | 20 x log10(P/P0) |
The symbol dB without one of the qualifiers listed above could imply that any pressure or intensity has been used as the reference.
Another important thing to know about deciBels is how to interpret values of different dB relative to each other. For example, a 6dB rise implies a doubling of sound pressure whilst a 3dB rise implies a doubling of intensity. Conversely, a 6dB fall implies a halving of sound pressure whilst a 3dB fall implies a halving of sound intensity.
| 20 x log10(2/1) | = | 6dB (SPL) |
| 20 x log10(0.5/1) | = | -6dB (SPL) |
| 10 x log10(2/1) | = | 3dB (IL) |
| 10 x log10(0.5/1) | = | -3dB (IL) |
where "2/1" implies that the new value (pressure in the first case and intensity in the second case) is twice the old value and "0.5/1" implies that the new value is half the old value. In all cases the "old" value here is equal to 1.
Adding or subtracting two dB or Sound Pressure values
You must be very careful when doing arithmetic on sound amplitudes. All calculations should be carried out on sound intensities, never on sound pressures or dB values (except for a small number of exceptions, and only when you are VERY clear about what you are doing).
For example, when adding together two sounds:-
| dB: |
dB1 + dB1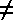 2 x dB1 2 x dB1 |
| Pressure: |
P1 + P1 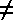 2 x P1 2 x P1 |
| Intensity: | I1 + I1 = 2 x I1 |
Only the addition or multiplication of intensities results in a correct answer. When two identical sounds are added together, the effect on dB and P values is as follows:-
| dB: | dB1 + dB1 = dB1 + 3 |
| Pressure: | P1 + P1 = 1.414 x P1 (1.414 = square root of 2) |
Since I  P2 then the doubling of intensity only results in an increase in pressure by 1.414. [ie. 2/1 = (1.414/1)2]. Further, as outlined in the previous section, doubling a sound results in an increase of 3 dB since the ratio of the new intensity to the old intensity is 2/1 and this results in a value of +3 dB.
P2 then the doubling of intensity only results in an increase in pressure by 1.414. [ie. 2/1 = (1.414/1)2]. Further, as outlined in the previous section, doubling a sound results in an increase of 3 dB since the ratio of the new intensity to the old intensity is 2/1 and this results in a value of +3 dB.
To add together two sound pressures, convert to intensities by squaring, add the squares together and the take the square root of the result, as in the following example where two sounds of P = 1 are added together (11):-
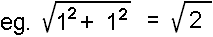 If you are asked to add together two sounds with known dB values and to determine the resultant amplitude in dB you must follow this procedure:-
If you are asked to add together two sounds with known dB values and to determine the resultant amplitude in dB you must follow this procedure:-
i) Convert both dB values to intensity (nb. it doesn't matter whether the dB values were derived from IL or SPL, the calculations MUST be performed on intensities).
Since:
dB = 10 x log10(I1/I0) then I1/I0 = 10(dB/10)
This formula can be simplified by arbitrarily assuming I0 = 1. This gives:-
I1 = 10(dB/10)
ii) Add together the derived intensity values:-
I = I1 + I2
iii) Convert the intensity back to dB (remembering to utilise the same reference I0 = 1 that was used in the first calculation, thus again removing I0 from the calculation):-
dB = 10 x log10(I)
This procedure can be simply extended to deal with the addition of more than 2 sounds or the subtraction of one sound from another.
Some tricks that may simplify dB calculations
i) When adding together two sounds of the same dB value, simply add 3 dB
ii) When doubling intensity, simply add 3 dB (ie. same as i) )
iii) When halving intensity, simply subtract 3 dB
iv) Multiplying intensity by four is the same as doubling twice, so add 3 dB twice, etc.
v) Dividing intensity by four is the same as halving twice, so subtract 3 dB twice, etc.
vi) When adding together two sounds the resultant dB value is somewhere between the higher original dB value and 3 dB above that value. That is for two sounds of dB1 and dB 2 (where the higher value is dB1) the resultant value is between dB 1 and dB 1+3. The new value is only equal to dB1+3 when dB2 exactly equals dB1. If your result is outside this range then your calculations are wrong.
| eg. | 50 dB + 50 dB = 53 dB |
| 50 dB + 47 dB = 51.8 dB | |
| 50 dB + 40 dB = 50.4 dB | |
| 50 dB + 20 dB = 50.004 dB | |
| 50 dB + 0 dB = 50.00004 dB (nb. 0 dB is not I = 0) |
Root Mean Squared (RMS) Average of Sound Pressure
The R.M.S. method is the only valid way to determine the "average" sound pressure of a length of speech signal. This is because pressures cannot be added together in a straightforward way but must be first converted to intensities. It must be remembered that:-
I  P2
P2
This implies that squaring P will effectively convert it into an intensity value of arbitrary units (dividing by 202 would convert it to standard units but this is unnecessary as the 20 2 factor would be cancelled out in the later reconversion to pressure). Therefore, the following formula can be used for the calculation of average pressure:-
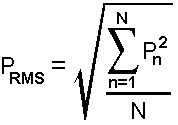
Average intensity can, on the other hand, be derived as a simple mean or average:-
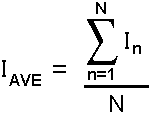
The table below demonstrates how to calculate the R.M.S. average of a set of five (N=5) pressure values. The first column indicates the value of "n" (ie. this line refers to the 1st, 2nd, ... 5th pressure value. The second column is the actual pressure value (eg. P2=2). The third column is the square of the pressure value. The 6th line of the 3rd column is the sum of the squared values. The 7th line is the R.M.S. value which is calculated by dividing the "SUM OF THE SQUARES" by the number of values (N=5) and then taking the square root of the result. In other words, the R.M.S. value is the:-
SQUARE ROOT OF (THE AVERAGE OF (THE SUM OF (THE SQUARES OF (THE ORIGINAL PRESSURE VALUES)))).
| n | Pn | Pn2 |
| 1 | 3 | 9 |
| 2 | 2 | 4 |
| 3 | 0 | 0 |
| 4 | -2 | 4 |
| 5 | -3 | 9 |
 Pn2 Pn2 | 26 | |
| PRMS | 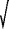 (26/5) (26/5) | |
Acoustic Intensity and the Inverse Square Law(12)
The acoustic intensity, or average rate at which work is being transferred through a unit area (on the surface of the spherical wave front radiating out from the source in all directions) diminishes with distance in accordance with the inverse square law.
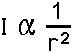
| where:- |
I  the intensity of a sound the intensity of a sound
|
r  the distance from the source of the sound the distance from the source of the sound
|
For the purposes of this course, we are mostly interested in comparing intensities (and pressures) at varying distances from the sound source. Use the following formula:-
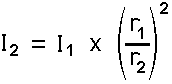
| where:- | I1 the intensity of a sound at distance r1 from the source |
| I2 the intensity of the same sound at distance r2 from the source. |
If you are asked the effect of increasing the distance by multiplying by a factor of X, the ratio on the right hand side of the last equation becomes (1/X)2 and so the value of I2 is simply I1 multiplied by (1/X)2. For example, if the new distance r2 is twice the old distance r1 from the sound source, then the new intensity I2 is (1/2)2 or 1/4 times the old intensity I1. You don't need to know what the actual distances are if the distances are referred to in any question as a ratio because the above equation is expressed as a ratio of 2 distances and of 2 intensities.
Q. If the intensity at a certain distance from a sound's source is 25 W.m-2, what is the intensity if the distance is increased five-fold ([r2/r1] = 5, so [r1/r2] = 1/5).
A. I2 = I1 x (r1/r2)2 = 25 x (1/5)2 = 25 x (1/25) = 1 W.m-2
Q. If the intensity at a certain distance from a sound's source is 20 W.m-2, what is the intensity if the distance is decreased by one fifth ([r2/r1] = 1/5, so [r1/r2] = 5).
A. I2 = I1 x (r1/r2)2 = 20 x (5)2 = 20 x (25) = 500 W.m-2
It can also be readily shown that:-
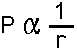
So, if we double the distance from the source we only halve the sound pressure (although we divide the intensity by four).
Q. If the sound pressure at a certain distance from a sound's source is 25 Pa, what is the sound pressure if the distance is increased five-fold ([r2/r1] = 5, so [r1/r2] = 1/5).
A. P2 = P1 x (r1/r2) = 25 x (1/5) = 5 Pa.
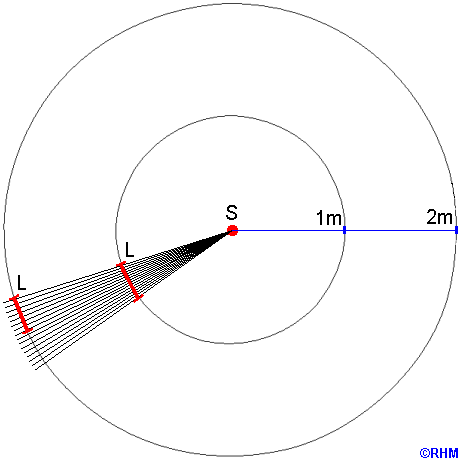
Figure 1: A two dimensional simulation of the inverse square law.
Figure 1 simulates the equivalent of the inverse square law in a 2 dimensional universe. In this diagram equal amounts of sound intensity are represented by each of the radial lines. At distance 1m 16 lines pass through an arc of length L. At distance 2m only 8 lines pass through an arc of length L and the 16 lines now pass through an arc of length 2L. This means that in a 2 dimensional universe sound intensity halves every time distance from the source is doubled (ie. an inverse law rather than an inverse square law).
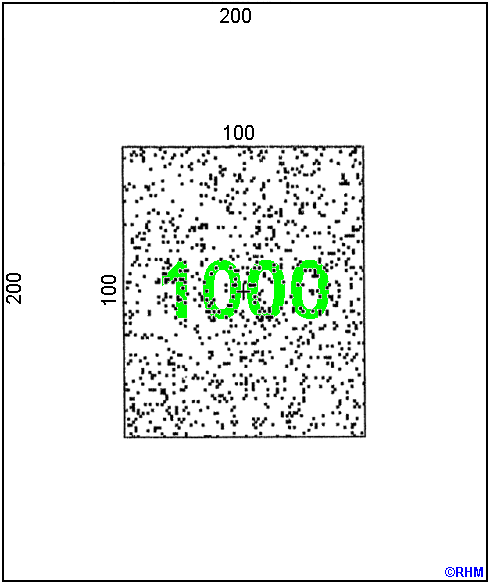
Figure 2: A three dimensional simulation of the inverse square law at distance R.
Figure 2 simulates the inverse square law in a 3 dimensional universe. In this diagram the squares represent areas on the surface of a sphere at a distance R from a sound source in the centre of the sphere. The dots are the equivalent of the lines in figure 1 and indicate the points at which 1000 lines (of sound intensity) pass through the 100 unit sided square on the surface of the sphere.
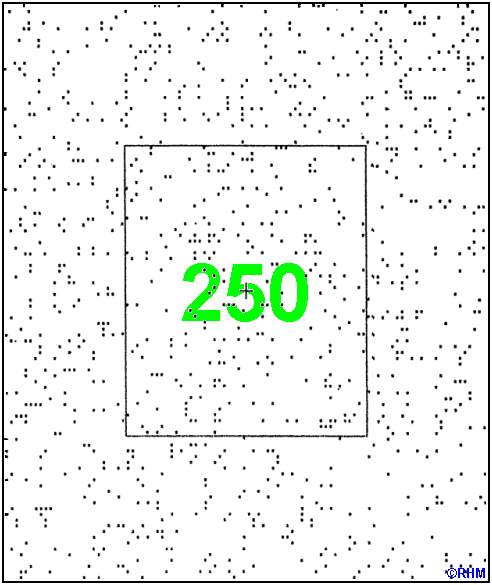
Figure 3: A three dimensional simulation of the inverse square law at distance 2R.
Figure 3 simulates the inverse square law in a 3 dimensional universe. In this diagram the squares represent areas on the surface of a sphere at a distance 2R from a sound source in the centre of the sphere. The dots are the equivalent of the lines in figure 1 and indicate the points at which 1000 lines (of sound intensity) pass through the 200 unit sided square on the surface of the sphere. Note, however that only 250 intensity lines pass through the inner 100 unit sided square. This is one quarter the number of intensity lines that pass through the 100 unit sided square at half this distance (ie. at distance R) from the central sound source. At twice the distance the length of each side of the square containing a fixed measure of sound intensity has doubled (as happened for the arcs in the the 2 dimensional world in figure 1). Since each of the sides of this square doubles with a doubling of the distance from the centre, the size of the square has increased by 4. In other words, sound intensity diminishes in accordance with the inverse square law for a fixed surface area (such as the area of the ear's tympanic membrane or the area of the transducer surface of a microphone).
The Doppler Effect
When both a sound source (S) and an observer (O) are stationary, the frequency observed by O can be readily determined from the wavelength of the sound emitted by S and the speed of sound according to the following formula.
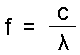
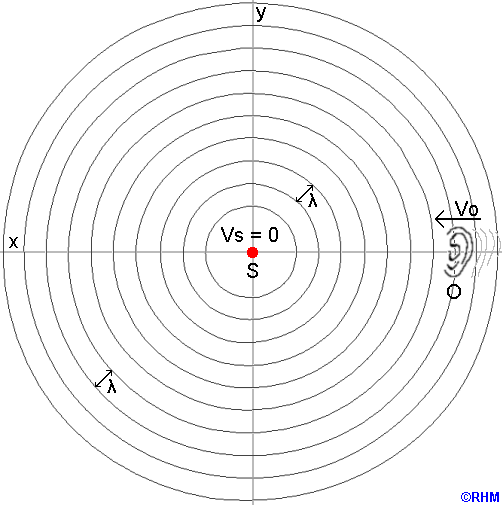
Figure 1: Illustration of the doppler effect with stationary sound source (S) and moving observer (O). The concentric circles represent the cycle peaks of the radiating sound waves.
When an observer (O) is moving towards a sound source (S), O's ear intersects with each cycle peak more rapidly than would be predicted from the wavelength and the speed of sound. This has the same effect as would an increase in the speed of sound. That is, there is an increase in the observed frequency of the sound. The reverse occurs when the observer is moving away from the sound (ie. it takes longer for each cycle peak to reach O's ear and so the observed frequency is lower). In these cases the effective speed of sound can be determined by adding Vo to c when O is moving towards the sound and by subtracting Vo from c when O is moving away from the sound.
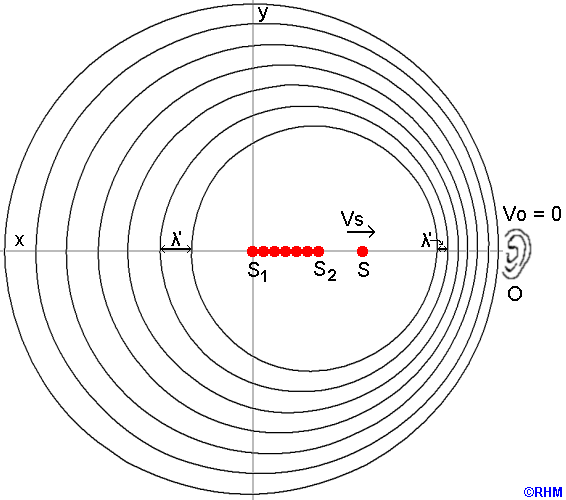
Figure 2: Illustration of the doppler effect with moving sound source (S) and stationary observer (O).
When a sound source (S) is moving towards an observer (O) each wave cycle is initiated a bit closer to O than was the preceding cycle. In this diagram the 7 dots between S1 and S2 represent the position of the source at the time of the propagation of the 7 wave cycles represented by the 7 circles. This has the effect of reducing the wavelength in the direction that S is moving. Reducing the wavelength increases the observed frequency of the sound. The reverse is true for an observer that the sound source (S) is moving directly away from. (Note that whilst this actually effects the sound's effective wavelength, the observed frequency of the sound can be more easily determined by adding Vs to c when S is moving towards O and by subtracting Vs from c when S is moving away from O.)
Footnotes
1. Some authors seem to use the term "amplitude" as a synonym of "pressure" (ie. "sound pressure level" - SPL). Other seem to use the term both generically and as a synonym for "sound pressure".
2. The vibrating air particles cause the microphone diaphragm to move in and out and sound pressure can be determined if the acceleration of the diaphragm is measured and the mass and area of the diaphragm are known. Microphones can be calibrated so that SPL can be measured directly.
3. See appendix 3 and appendix 4 for detailed descriptions of the Basic Physical Units of Measurement and specifically of the units of measurement of particular relevance to acoustics. In these notes the MKS system of measurement is used, and this is described in these appendices. In many older publications you may find reference to the now discarded CGS system of units and even to the British Imperial system of units. The CGS system is also described in the appendices.
4. The basic units of length (metre m), time (second s), and mass (kilogram kg) form the basis of most other units of measurement and of all common acoustic units of measurements.
5. See appendix 6 for a detailed explanation of the speed of sound in different media and conditions.
6. 1 atmosphere = 1.013 x 106 dynes.cm-2 in the CGS system
7. Threshold sound pressure level is 2 x 10-4 dynes.cm-2 in the CGS system
8. Threshold acoustic intensity level is 10-16 Watts.cm-2 in the CGS system
9. This is not strictly correct as the dB is a ratio, and so is not made up of basic units of measurement (ie. Intensity kg.s-3 whilst the dB is not)
10. See appendix 5 for the derivation of the formula for calculating dB from sound pressure level.
11. See the next section on RMS for more details.
12. See appendix 7 for further information on the inverse square law, including derivations of the formulae.
Content owner: Department of Linguistics Last updated: 12 Mar 2024 10:12am